Found on the internet - I can't explain it...                                                                                                                                           

Regular Tunings

A tuning $\tau(\mbox{\boldmath$x$})$ is regular if and only if it is a homomorphism, i.e. for all pitches x and y,

\begin{displaymath} \ensuremath{\ensuremath{\underline{\tau}}_{{}}\!\left(\mbox{... ...math{\underline{\tau}}_{{}}\!\left(\mbox{\boldmath$y$}\right)}.\end{displaymath}

This is a rather nice mathematical definition, but musically, what does it mean? Musically, a regular tuning is one in which an interval is tuned the same no matter where it occurs. Let us see how this flows from the mathematical definition of regularity. First of all, what does it mean to add pitches? When two pitches are added, think of the second one as an interval, so for example, $\mbox{\boldmath$x$} + \mbox{D0}$ becomes $\mbox{\boldmath$x$} + \mbox{M2}$. In a regular diatonic tuning $\tau(\mbox{\boldmath$x$})$,

\begin{displaymath} \ensuremath{\ensuremath{\underline{\tau}}_{{}}\!\left(\mbox{... ...th{\ensuremath{\underline{\tau}}_{{}}\!\left(\mbox{D0}\right)}.\end{displaymath}

No matter what x is, it has the same frequency ratio to the pitch an M2 above it. For example, the M2 from C3 to D3 is the same as that from D3 to E3. By identical means, it can be seen that all intervals of a given type are tuned the same no matter where they occur. The definition of a regular tuning above is similar to that of Lindley and Turner-Smith [14, 43] and Regener [20, 87-8].

For regular tunings, \ensuremath{\ensuremath{\underline{\Phi}}_{{}}\!\left(x\hspace{-1pt}_f\right)} and \ensuremath{\ensuremath{\underline{\Theta}}_{{}}\!\left(x\hspace{-1pt}_f\right)} are constants. In other words, all P5 deviate from just by the same amount, and all M3 deviate from just by the same amount. This is a natural consequence of the fact that in a regular tuning, all intervals of a given type are tuned the same no matter where they occur. Since for regular tunings $\Phi(x\hspace{-1pt}_f)$ and $\Theta(x\hspace{-1pt}_f)$are constant, we shall often abbreviate them as $\Phi$ and $\Theta$.These are similar to Lindley and Turner-Smith's $t_{\text{V}}$ and $t_{\text{III}}$ respectively. We shall see how to calculate these amounts below.

12TET is an example of a regular diatonic tuning. In fact, it is the only regular diatonic tuning that can be implemented on an instrument with 12 frequencies per doubling, as will be shown later.

A regular diatonic tuning $\tau(\mbox{\boldmath$x$})$ has a regular fifth tuning underlying it. Let us see why this is true. Since $\tau(\mbox{\boldmath$x$})$ is regular for all pitches x and y, this certainly applies to all pitches in register zero. By definition, the register-zero tuning is doing all the tuning of register zero, so it, too, is regular. Since all intervals of the same type are tuned the same in a regular tuning, its fifth tuning can be characterized by a single constant, v, the frequency ratio of P5. Since, in addition, we know that $\ensuremath{\ensuremath{\underline{\phi}}_{{}}\!\left(0\right)}=0$ (Eq. 3.1), we can state that regular tunings have the simple form  

   \begin{displaymath} \ensuremath{\ensuremath{\underline{\phi}}_{{}}\!\left(x\hspace{-1pt}_f\right)} = x\hspace{-1pt}_f\ensuremath{\underline{v}}.\end{displaymath} (4)

The values of \ensuremath{\underline{\Phi}} and \ensuremath{\underline{\Theta}} for a regular tuning can be calculated as follows:
 \begin{align} \ensuremath{\underline{\Phi}} &= \ensuremath{\underline{v}} - \ens... ... &= \ensuremath{\underline{C_S}} + 4\ensuremath{\underline{\Phi}}. &&\end{align}
We will now present some theoretically important regular tunings using the formulas developed above.

In Pythagorean tuning, all P5 are just, meaning $\ensuremath{\underline{\Phi}} = 0$and therefore

\begin{displaymath} \ensuremath{\underline{\Theta}} = \ensuremath{\underline{C_... ...Phi}} = \ensuremath{\underline{C_S}} \approx 17.9 \mbox{ mil}.\end{displaymath}

In 1/4-comma meantone (QCM) tuning, all M3 are just, meaning $\ensuremath{\underline{\Theta}} = 0$ and therefore

\begin{displaymath} \ensuremath{\underline{\Phi}} = (\ensuremath{\underline{\Th... ...)/4 = -\ensuremath{\underline{C_S/4}} \approx -4.5 \mbox{ mil}\end{displaymath}

We can see that the comma referred to in the name of this tuning is the syntonic comma.

In 12TET, all d2 are unisons, meaning $\ensuremath{\ensuremath{\underline{\tau}}_{{}}\!\left(\mbox{d2}\right)} = 0$. Since d2 = \ensuremath{[-12\ {7}]^\mathrm{T}},
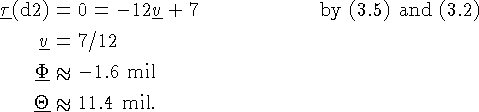
\begin{align*} \ensuremath{\ensuremath{\underline{\tau}}_{{}}\!\left(\mbox{d2}\r... ...} &&\\ \ensuremath{\underline{\Theta}}&\approx 11.4 \mbox{ mil}. &&\end{align*}
In 1/5-comma meantone (FCM) tuning, P5 are flat (from just) by the same amount M3 are sharp (from just). This has an appealing egalitarianism to it.
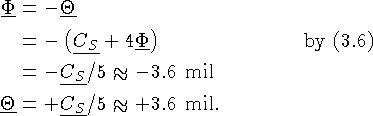
\begin{align*} \ensuremath{\underline{\Phi}} &= -\ensuremath{\underline{\Theta}}... ...Theta}} &= +\ensuremath{\underline{C_S}}/5 \approx +3.6 \mbox{ mil}.\end{align*}
Once again, we see that the comma referred to in the name of this tuning is the syntonic comma.

Figure 3.9 compares the regular tunings presented above in terms of $\Phi$ and $\Theta$. In addition, it shows the line $\ensuremath{\underline{\Theta}} = \ensuremath{\underline{C_S}} + 4\ensuremath{\underline{\Phi}}$ on which all regular tunings lie.


 

  
Figure 3.9: Comparison of regular tunings
\begin{figure} \setlength {\unitlength}{10pt} \centering \begin{picture} (... ...5){\vector(4,1){19}} \put( 0, -4.5){\vector(-4,-1){1}}\end{picture}\end{figure}

 

The Size of Regular Diatonic Tunings

The size of a tuning is an important factor if it is to be implemented on an instrument that can produce only a small number of frequencies per doubling, like the piano, which can only produce 12. The size of a tuning is the number of unique values $\ensuremath{\ensuremath{\underline{\phi}}_{{}}\!\left(x\hspace{-1pt}_f\right)} \bmod 1$ takes on. For example, 12TET has size 12 since $\ensuremath{\ensuremath{\underline{\phi}}_{{}}\!\left(x\hspace{-1pt}_f\right)} \bmod 1$ is $(7/12)x\hspace{-1pt}_f\bmod 1$, which takes on only 12 unique values. The size of a tuning is also the number of frequency ratios per doubling that is required to implement it. Most regular tunings are very large (many even infinite!) and therefore difficult or impossible to implement on an instrument with a finite number of frequencies per doubling.

Regular tunings with rational \ensuremath{\underline{v}} equal to some fraction n/k have size k, assuming n/k is in reduced form. These are the equal temperaments, like 12TET and 19TET ($\ensuremath{\underline{v}}=11/19$). A regular tuning with an irrational \ensuremath{\underline{v}} has infinite size. Mathematically, this means that $\tau(\mbox{\boldmath$x$})$ is a one-to-one mapping. By Eq. 3.5 and Eq. 3.2,

\begin{displaymath} \ensuremath{\ensuremath{\underline{\tau}}_{{}}\!\left(\mbox{... ...ht)} + x_r = x\hspace{-1pt}_f\ensuremath{\underline{v}} + x_r. \end{displaymath}

The function $\tau(\mbox{\boldmath$x$})$ is one-to-one if and only if any change to x results in a different value yielded. Clearly a change to $x\hspace{-1pt}_f$ or xr alone will result in a different value. In addition, any change to $x\hspace{-1pt}_f$ changes the value of $\tau(\mbox{\boldmath$x$})$ by an irrational amount, so there is no way to cancel this change with a change to xr since xr is an integer.

We will sometimes refer to a tuning of size n as an n-tuning.

Hit CounterFrom Jan 1, 2007
Back to Interesting Things